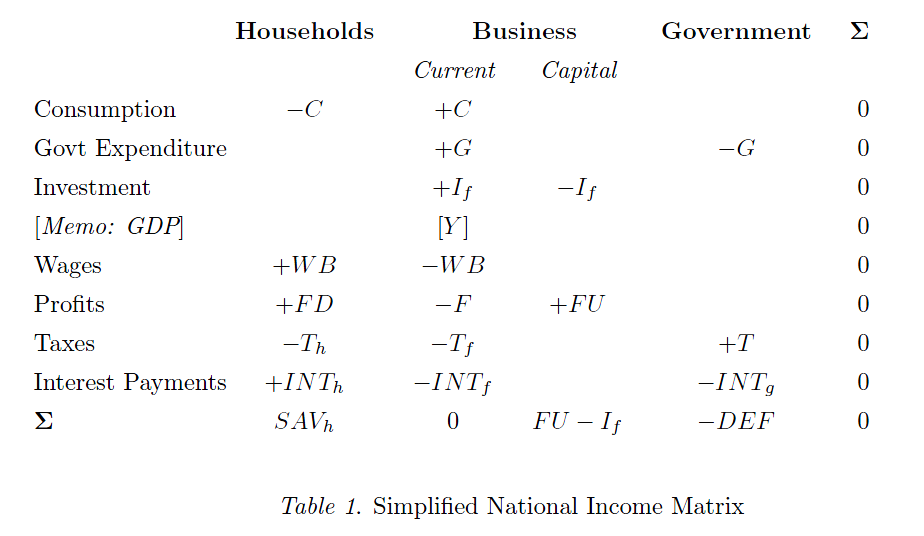
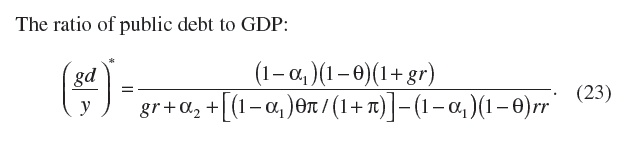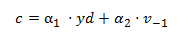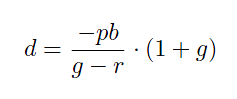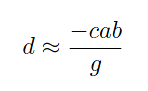Tag Archives: G&L

The α2 Parameter In Stock-Flow Consistent Models

Posted on by
Leave a reply

Let us think of a closed economy. Assuming – every year the government runs a budget deficit – a careless analysis would imply the public debt keeps rising relative to gdp.

Without going into derivation – which you can find in other sources – it can be shown that if there is a growth rate of g, the public debt converges to

GD/Y = (PDEF/Y)/(g – r)

where GD is the government debt, Y – the national income, PDEF – the primary government deficit (government expenditure excluding interest payments less tax revenues) and g and r are the nominal growth rates and the interest rate respectively.

Several things. The above assumes – implicitly – that DEF is a constant percent of GDP (Y). Second, it neglects the fact that interest income is also income to bond holders which leads to more consumption. Third, less importantly, it ignores taxes on interest income. The first one is important. There is an implicit assumption of the exogeneity of the budget deficit and the above expression has nothing to say about the private sector’s propensity to save, consume etc.

The above expression has been derived using the assumption that g > r and summing a series. For the opposite case, the series used to derive it diverges. [For example, the equation

1 + x + x2 + x3 + … = 1/(1 – x)

is valid only if x < 1. For x > 1, the left hand side diverges and not negative as the formula implies!]

This has led authors to argue that if the growth rate is higher than the average interest rate paid on government debt, then the public debt doesn’t rise forever.

This intuition is not so right – although there is an element of truth in it but needs to be used extremely carefully.

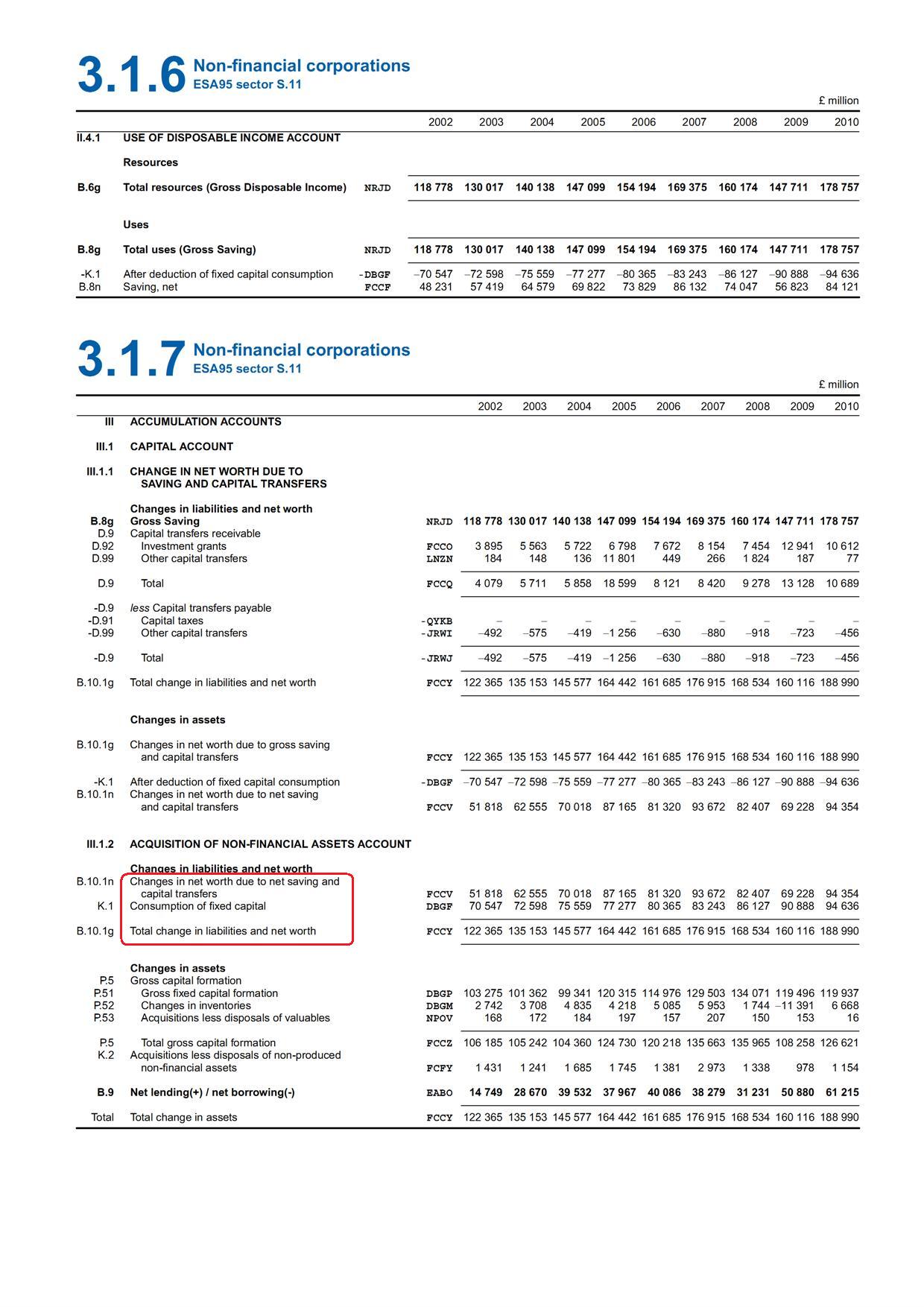
Wynne Godley and Marc Lavoie [1] (but also [2] – which I haven’t managed to get hold of) show what this “stock-flow norm” converges asymptotically in the case of a very simple model of a closed economy – even when the government is not targeting a primary surplus in the budget.  The following are from their paper and the lower case is used to denote real variables instead of nominal: 

In the above gd is the real government debt, y is the real national income, α1 is the propensity of households to consume out of disposable income, α2 is the propensity to consume out of wealth, gr is the growth rate of output, rr is the real interest rate, π  is the rate of inflation and θ is the tax rate.

The assumed consumption function is:

where c is real consumption,  yd is household real disposable income, and v is real household wealth.

 This expression gives some intuition. The growth rate can be quite less than the interest rate and yet the public debt can be bounded. This is because bond interest payments by the government is income for bond holders. More importantly, the denominator contains α2 which significantly brings the (public debt/gdp) ratio down (compared to the case where α2 is zero).

Looking at the model and how the variables change, one can conclude that the government needn’t pursue a policy of targeting a primary surplus, contrary to the intuition neoclassical economists obtain by doing debt sustainability analysis. The budget may reach a primary surplus automatically as a result of higher taxes due to higher activity.

Also, there is no condition “g > r”. 

One may wonder what value the parameter α2 has for various economies. According to Lance Taylor – a reviewer of Wynne Godley’s work [3] –  the value could be 0.04 or 4% from econometric studies but he does notice the tendency of G&L to choose a higher value in pedagogic examples.

In my opinion it is higher. I think it’s a good challenge to try to show this empirically. This may be true because (abstracting out the effect of the external sector, the public debt ratio is better explained and also that a high α2 implies a quick response to a fiscal expansion – which is true.

One should be highly careful about debt sustainability analysis. For the case of an open economy, while it is true that a debtor nation can be a debtor forever under some assumptions, achieving a faster growth in a world of free trade can lead to stock/flow ratios rising forever instead of converging. Which is to say that nations are balance-of-payments constrained.

References

  1. Wynne Godley and Marc Lavoie, Fiscal Policy In A Stock-Flow Consistent Model, p 79, Journal of Post Keynesian Economics / Fall 2007, Vol. 30, No. 1. Draft version available at http://www.levyinstitute.org/publications/?docid=911
  2. Wynne Godley and Bob Rowthorn, Appendix; The Dynamics Of Public Sector Deficit And Debt, in J. Michie and J. Grieve Smith (eds), Unemployment in Europe (London: Academic Press), pp. 199-206.
  3. Lance Taylor, A Foxy Hedgehog: Wynne Godley And Macroeconomic Modelling, Camb. J. Econ. (2008) 32 (4):639-663.

More On Wynne Godley’s Methodology

Posted on by
Leave a reply

Matias Vernengo has a post on Stock-Flow Consistent Macroeconomics: Stock-Flow With Consistent Accounting (SFCA) Models.

He has a nice way of giving a short description of pricing in the G&L models:

In my view, the stock-flow and the demand driven (and I should say, the fact that price dynamics is orthogonal to the income flow determination structure) is the essential characteristic of this approach.

—

Also, Simon Wren-Lewis (from Oxford) has a new blog post on the sectoral balances approach – Sector Financial Balances As A Diagnostic Check, where he mentions Martin Wolf’s recent post on Wynne Godley’s approach. He (Wren-Lewis) has been admitting recently that DSGE models are not useful.

In the comments section Simon Wren-Lewis has this to say:

Martin Wolf sent me the following comment, which I am sure others will also find interesting:

“I used sectoral financial balances before the crisis, following Wynne. I argued that what was going on in the US external and household sectors were evidently unsustainable. This allowed me to argue that when the latter’s deficits were eliminated, there would be a recession and a huge fiscal deficit. What I had not expected was that the turnaround in the household sector would trigger a meltdown of the financial system.

“This makes it clear that one has to link the flow sectoral balances to the balance sheets in the economy. In this case, my mistake was not looking closely enough at the balance sheet of the financial sector. Good macroeconomic analysis has to examine the flows and stock meticulously and seek to assess whether the behaviour we see is sustainable. The assumption that private agents cannot make huge mistakes about the sustainability of what they are doing is, in my view, the biggest mistake in macroeconomics.”

Back to DSGE models. I think they are totally useless. I like this quote by Francis Cripps from an article in The Guardian from 27 Feb 1979: Economists With A Mission:

 

Philip Pilkington Interviews Marc Lavoie

Posted on by
Leave a reply

Philip Pilkington’s interview of Marc Lavoie on his textbook Monetary Economics is available to read at Naked Capitalism. New Directions in Monetary Economics: An Interview with Marc Lavoie – Part I

Part 2 appears here: New Directions in Monetary Economics: An Interview with Marc Lavoie – Part II

Wynne Godley used to think that Keynesians were being defeated by Monetarists because they (the Keynesians) simply could not answer how money (in general assets and liabilities) is created and hence wrote the book Macroeconomics with Francis Cripps. After that he wanted to make it even more solid.

Marc Lavoie says:

… it is clear that Wynne wished to depart from neoclassical economics, and start from scratch, which is what he did to some extent already when Wynne and his colleague Francis Cripps wrote a highly original book that was published in 1983, Macroeconomics. This book was written because Wynne got convinced that the Keynesians of all strands were losing their battle against Milton Friedman and the monetarists, because Keynesians could only provide very convoluted answers to simple questions such as: “Where does money come from? Where does it go? How do the income flows link up with the money stocks? How is new production financed?”

Our book Monetary Economics also tries to provide appropriate answers to these questions. We agonized for a while between trying to engage in a constructive dialogue with our mainstream colleagues and targeting a non-mainstream audience, or perhaps trying to achieve both goals. In the end, we figured that it would be very difficult to please both audiences, and we chose to focus on a heterodox audience. In any case, I have spent most of my academic career trying to develop alternative views and alternative models of economics – what is now called heterodox economics; this is the literature we know best. So we took our book as a formal contribution to this heterodox literature and more specifically as a contribution to post-Keynesian economics.

Marc Lavoie at the Levy Institute, May 2011 (Photo Credits: me 😉 )

Balance Of Payments: Part 2 – Double Versus Quadruple Entry Bookkeeping

Posted on by
Leave a reply

Some time back I had started with the first part of a series of posts on this topic: see Balance Of Payments: Part 1. From the same post, here’s from the Australian Bureau of Statistics’ manual Balance of Payments and International Investment Position, Australia, Concepts, Sources and Methods, 1998

(click to enlarge)

So we have the current account, the financial and the international investment position at the beginning and end of each accounting period. In addition we have, revaluations on assets and liabilities. These arise due to change in the value of assets (such as rise in stock markets) and due to movement of the exchange rate or both.

Also, textbooks use a slightly different language than official statistics and manuals. Textbooks simply use the phrase capital account when they mean the financial account.

I aim to go into each of this and the behaviour of institutions who are involved in the whole process and how it leads to changes in assets and liabilities of all sectors and the consequences. We will see how endemic current account deficits act as a hemorrhage in the circular flow of national income as Wynne Godley would put it and decides the fate of nations as Anthony Thirlwall may have it.

To really appreciate, one needs to have a strong methodology for studying this. One way is to use G&L’s transactions flow matrix but it can get complicated in case of two nations. Needless to say, from a modeling perspective, it is more useful than the usual way of studying balance of payments. However, for appreciating G&L methodology one needs to first understand the usual way of studying this.

Double Entry Versus Quadruple Entry Bookkeeping

In contrast to national accounts, Balance of Payments is based on double entry bookkeeping. Here’s from the IMF’s Balance of Payments And International Investment Position Manual (BPM6), pg 9:

The balance of payments is a statistical statement that summarizes transactions between residents and nonresidents during a period. It consists of the goods and services account, the primary income account, the secondary income account, the capital account, and the financial account. Under the double-entry accounting system that underlies the balance of payments, each transaction is recorded as consisting of two entries and the sum of the credit entries and the sum of the debit entries is the same.

In contrast, national accounts as per SNA2008 or G&L’s way of doing it uses quadruple entry bookkeeping who point out in their book Monetary Economics that:

… Copeland pointed out that, ‘because moneyflows transactions involve two transactors, the social accounting approach to moneyflows rests not on a double-entry system but on a quadruple-entry system’. Knowing that each of the columns and each of the rows must sum to zero at all times, it follows that any alteration in one cell of the matrix must imply a modification to at least three other cells. The transactions matrix used here provides us with an exhibit which allows to report each financial flow both as an inflow to a given sector and as an outflow to the other sector involved in the transaction.

G&L point out that even Hyman Minsky was aware of this. Here’s from the article The Essential Characteristics of Post-Keynesian Economics (page 20):

The structure of an economic model that is relevant for a capitalist economy needs to include the interrelated balance sheets and income statements of the units of the economy. The principle of double entry book keeping, where financial assets are liabilities on another balance sheet and where every entry on   balance sheet has a dual in another entry on the same balance sheet, means that every transaction in assets requires four entries.

The System of National Accounts 2008 (2008 SNA) says (page 21):

In principle, the recording of the consequences of an action as it affects all units and all sectors is based on a principle of quadruple entry accounting, because most transactions involve two institutional units. Each transaction of this type must be recorded twice by each of the two transactors involved. For example, a social benefit in cash paid by a government unit to a household is recorded in the accounts of government as a use under the relevant type of transfers and a negative acquisition of assets under currency and deposits; in the accounts of the household sector, it is recorded as a resource under transfers and an acquisition of assets under currency and deposits. The principle of quadruple entry accounting applies even when the detailed from-whom-to-whom relations between sectors are not shown in the accounts. Correctly recording the four transactions involved ensures full consistency in the accounts.

Simple example: your and my favourite: loans make deposits. The following is a transaction where a household has borrowed some funds from the banking sector:

 

Introduction To Current Transactions

I mentioned that in recording transactions between residents and nonresidents and presenting it as balance of payments, national accountants use double entry bookkeeping (as opposed to quadruple), so any transaction in the current account necessarily involves another entry in the financial account (ignoring barter and accidental cancellations). However, the opposite is not the case: a transaction on the financial account will lead to another entry in the financial account and not directly in the current account. A purchase of US equities by a UK resident cannot be said to cause or increase the US current account deficit.

One example: if you are are US citizen travelling to the UK and have pay for coffee at the London airport by paying in Federal Reserve notes, it will give rise to an entry in the current account (credit from the perspective of the UK balance of payments) and a debit (increase in assets of UK residents: change in currency notes). This is just transaction among thousands and the question is how is all this to be recorded and more importantly (later) what does it tell us.

Here’s how a standard balance of payments table looks like (note: this does not include international investment position)

(source: UK Pink Book 2011; click to enlarge)

We will go over details in the next post in this series. For now let us see how this looks for the example presented earlier: A US traveller pays $10 for coffee at the London Heathrow airport with Federal Reserve currency notes. Assuming the current exchange rate, the following (double) entries need to be included in the UK balance of payments:

 

£ CreditsDebits
Current Account
Goods and Services6.328
Financial Account
Bank Deposits, Foreign Currency Assets6.328

 

 

This is a simple example – hardly needing so much background and information but in the next post in this series, we will look at complicated examples where intuitions can easily go wrong. If the above were the only transaction between UK and US residents in the accounting period (quarter/year), this will also change the US indebtedness to the UK by £6.328 or $10 and this will be shown in the international investment positions of the UK and the US. If the exchange rate had moved from the start of the period, revaluations would need to be done to record the closing stocks of assets and liabilities.

G&L’s Monetary Economics – Second Edition

Posted on by
Leave a reply

I got my copy of Monetary Economics by Wynne Godley and Marc Lavoie yesterday. I know some people were waiting for the second edition of the book, and had postponed their purchase to get the newer edition – so they can get it now!

There aren’t any changes in this edition – except for correction of some typos and that this edition is a paperback while the first one was hardcover. I already knew this as Marc Lavoie told me “don’t buy it” – but of course how can I not!

One thing I noticed is a nice summary by Wynne Godley which he wrote after the first edition was published.

Here’s an autograph from Marc Lavoie I got last year in May – live to tell!

I hope I live up to it 🙂

The first time I saw something called the Transactions Flow Matrix in a Levy Institute paper, I rushed to buy the book. When I started reading it, it became clear that nobody has ever come close to it! After a while – and solving the models on a computer gives one greater intuition – it slowly started becoming clear to me why so much effort has been put in.

Wynne Godley always wanted to write a textbook to help others understand Cambridge Keynesianism, as he often thought that while top economists from Cambridge knew how economies work together, they never attempted to share this knowledge. I think his aim was also to sharpen his own knowledge and to think of scenarios which one may not be able to foresee using simple arguments.

With this aim, he made a first attempt with his partner at “New Cambridge”, Francis Cripps.

I really like this from the book’s introduction:

… Our objective is most emphatically a practical one. To put it crudely, economics has got into an infernal muddle. This would be deplorable enough if the disorder was simply an academic matter. Unfortunately the confusion extends into the formation of economic policy itself. It has become pretty obvious that the governments of many countries, whatever their moral or political priorities, have no valid scientific rationale for their policies. Despite emphatic rhetoric they do not know what the consequences of their actions are going to be. Moreover, in a highly interdependent world system this confusion extends to the dealings of governments with one another who now have no rational basis for negotiation.

This was a great book but didn’t receive much attention except for a small group who thought (rightly!) it was a work of genius. He wanted to do more and so we see him mention in an article on him – praising his prescience on writing the fate of the British economy on the wall in the 70s and the 80s. Here’s from the Guardian:

… What I’m doing is abolishing economics as currently understood, conducting an enormous sanitary operation upon a very clogged profession. I’m going to push a dose of salts through that system. It is a ruthless application of logic and accountancy to macroeconomics.

(click to enlarge and click again)

When Wynne Godley lost the partnership of Francis Cripps (whom Wynne called the smartest Economist he ever met), he was forced to do a lot of things himself and probably felt somewhat alone. After writing some amazing papers in the 1990s, he needed someone like Cripps to write a book. Fortunately he met Marc Lavoie and they collabrated for many years in writing papers and articles and finally the book.

Marc Lavoie recalls the memories in this article from the Godley conference last year.

In an article A Foxy Hedgehog: Wynne Godley And Macroeconomic Modelling – reviewing the book and Wynne Godley’s models, Lance Taylor says:

The fox knows many things, but the hedgehog knows one big thing.

… Surely someone who puts so much effort into one complicated construct is more like Plato than Aristotle, Einstein than Feynman, Proust than Joyce.

Kaldor’s Reflux Mechanism

Posted on by
Leave a reply

The recent debates of Post Keynesians with Neoclassical/New Keynesians has highlighted that the latter group continues to hold Monetarists’ intuitions. Somehow the exogeneity of money is difficult for them to get rid of, in spite of their statements and rhetoric that money is endogenous in their models.

So there is an excess of money in their models and this gets resolved by a series of buy and sell activities in the “market” (mixing up decisions of consumption and portfolio allocation) until a new “equilibrium” is reached where there is no excess money.

Two-Stage Decision

While the following may sound obvious, it is not to most economists. Keynes talked of a two-stage decision – how much households save out of their income and how they decide to allocate their wealth. In the incorrect “hot potato” model of Neoclassical economists and their New “Keynesian” cousins, these decisions get mixed up without any respect for the two-stage decision. It is as if there enters an excess money in the economy from somewhere and people may consume more (as if consumption is dependent on the amount of deposits and not on income) till prices rise to bring the demand for money equal to what has been “supplied” (presumably by the central bank).

In their book Monetary Economics, Wynne Godley and Marc Lavoie have this to say (p 103):

A key behavioural assumption made here, as well as in the chapters to follow, is that households make a twostage decision (Keynes 1936: 166). In the first step, households decide how much they will save out of their income. In the second step, households decide how they will allocate their wealth, including their newly acquired wealth. The two decisions are made within the same time frame in the model. However, the two decisions are distinct and of a hierarchical form. The consumption decision determines the size of the (expected) end-of-period stock of wealth; the portfolio decision determines the allocation of the (expected) stock of wealth. This behavioural hypothesis makes it easier to understand the sequential pattern of household decisions.

[Footnote]: In his simulation work, but not in his theoretical work, Tobin endorsed the sequential decision that has been proposed here: ‘In the current version of the model households have been depicted as first allocating income between consumption and savings and then making an independent allocation of the saving among the several assets’ (Backus et al. 1980: 273). Skott (1989: 57) is a concrete example where such a sequential process is not followed in a model that incorporates a Keynesian multiplier and portfolio choice.

Constant Money?

So even if one agrees that loans make deposits, there is still a question of deposits just being moved around and the (incorrect) intuition is that someone somewhere must be holding the deposit and hence similar to the hot potato effect. The error in this reasoning is the ignorance that repayment of loans extinguishes money (meant to be deposits in this context).

Nicholas Kaldor realized this Monetarist error early and had this to say

Given the fact that the demand for money represents a stable function of incomes (or expenditures), Friedman and his associates conclude that any increase in the supply of money, however brought about (for example, through open-market operations that lead to the substitution of cash for short-term government debt in the hands of discount houses or other financial institutions), will imply that the supply of money will exceed the demand at the prevailing level of incomes (people will “find themselves” with more money than they wish to hold). This defect, in their view, will be remedied, and can only be remedied, by an increase in expenditures that will raise incomes sufficiently to eliminate the excess of supply over the demand for money.

As a description of what happens in a modern economy, and as a piece of reasoning applied to situations where money consists of “credit money” brought about by the creation of public or private debt, this is a fallacious piece of reasoning. It is an illegitimate application of the original propositions of the quantity theory of money, which (by the theory’s originators at any rate) were applied to situations in which money consisted of commodities, such as gold or silver, where the total quantity in existence could be regarded as exogenously given at any one time as a heritage of the past; and where sudden and unexpected increases in supply could occur (such as those following the Spanish conquest of Mexico), the absorption of which necessitated a fall in the value of the money commodity relative to other commodities. Until that happened, someone was always holding more gold (or silver) than he desired, and since all the gold (and silver) that is anywhere must be somewhere, the total quantity of precious metals to be held by all money-users was independent of the demand for it. The only way supply could be brought into conformity, and kept in conformity, with demand was through changes in the value of the commodity used as money.

[boldening: mine]

from Nicholas Kaldor wrote a major article in 1985 titled How Monetarism Failed (Challenge, Vol. 28, No. 2, link).

In his essay Keynesian Economics After Fifty Years, (in Keynes And The Modern World, ed. George David Norman Worswick and James Anthony Trevithick, Cambridge University Press, 1983), Kaldor wrote:

The excess supply would automatically be extinguished through the repayment of bank loans, or what comes to the same thing, through the purchase of income yielding financial assets from the banks.

Here’s the Google Books preview of the page:

click to view on Google Books

In his article, Circuit And Coherent Stock-Flow Accounting, (in Money, Credit, and the Role of the State: Essays in Honour of Augusto Graziani, 2004. Google Books link) Marc Lavoie showed how this precisely works using Godley’s transactions flow matrix. (Paper available at UMKC’s course site). See Section 9.3.1

The fact that the supply of credit and demand for money appeared to be independent

 … has led some authors to claim that there could be a discrepancy between the amount or loans supplied by banks to firms and the amount or bank deposits demanded by households. This view of the money creation process is however erroneous. It omits the fact that while the credit supply process and the money-holding process are apparently independent, they actually are not, due to the constraints or coherent macroeconomic accounting. In other words, the decision by households to hold on to more or less money balances has an equivalent compensatory impact on the loans that remain outstanding on the production side.

So if households wish to hold more deposits, firms will have to borrow more from banks. If households wish to hold less deposits, they will purchase more equities (in the model) and hence firms will borrow lesser from banks and/or retire their debt toward banks.

Other References

  1. Lavoie, M. 1999. The Credit-Led Supply Of Deposits And The Demand For Money – Kaldor’s Reflux Mechanism As Previously Endorsed By Joan Robinson, Cambridge Journal Of Economics. (journal link)
  2. Kaldor N. and Trevithick J. 1981. A Keynesian Perspective On Money, Lloyd’s Bank Review.

More National Accounts: Consumption Of Fixed Capital

Posted on by
Leave a reply

In one of my recent posts, Saving Net Of Investment, I went into gross saving versus saving net of consumption of fixed capital. I showed how depreciation – or more appropriately, consumption of fixed capital – is treated in the flow of funds accounts.

Since the transactions flow matrix is a powerful tool for visualizing flow of funds, the question is where depreciation makes an appearance. The following table created by me using shows how for a simple economy.

FIGURE 1. Transactions Flow Matrix (click to enlarge)

Here “Firms” is a shorthand for all production firms as a sector and I took the consumption of fixed capital of firms only for illustration purposes. (Else I would have needed to break the households’ accounts into current and capital accounts – eating up space).

Investment here is gross investment and consumption of fixed capital makes its appearance in the line 3. It is a negative item in the current account and a positive item in the capital account. So it more of a book-keeping device but an important one because depreciation is not unimportant. The definition of profits is that of Wynne Godley and is slightly different from National Accounts. Also, while undistributed profits is a source of funds, CFC is also!

In my posts Net Worth and Net Worth: Part 2, I went into how net worth is defined. Also for a background on sources and uses of funds, see this post Sources And Uses Of Funds.

So undistributed profits (FU) and consumption of fixed capital (CFC) are both sources of funds. (Positive signs denote sources of funds and negative – uses of funds). This can be confusing because depreciation is a negative for net worth. The reason is that, as I have mentioned before, revaluations need to be done before end of period stocks are calculated. And it is where consumption of fixed capital will make a reappearance – subtracting from net worth due to a reduction in the value of nonfinancial assets.

It is important to keep in mind that equities are also sources of funds as the last line (above Σ) shows. So net saving (undistributed profits for firms) and consumption of fixed capital add to changes in net worth. (Note: Net is net of consumption of fixed capital here and not net of investment!).

This can be seen from the UK Blue Book 2011.

FIGURE 2.  UK Blue Book 2011 Accumulation Accounts (click to enlarge)

A Digression

What is the origin of the confusing phrase “net saving” – saving net of investment? I believe it came from Nicholas Kaldor himself who originated the sectoral balances approach. Here’s from The Scourge Of Monetarism, 1982, pp 48-50:

The PSBR in any year can be defined as the public sector’s net de-cumulation of financial assets (net dissaving) which by accounting identity must be equal to the net acquisition of financial assets (net saving) of the private sector, home and overseas; which in turn can be broken down to the net acquisition of financial assets of the personal sector, of the company sector, and the overseas sector (the latter is the negative of the balance of payments on current account).

Debt Sustainability

Posted on by
Leave a reply

In a recent paper, Bradford DeLong and Lawrence Summers suggest that a fiscal expansion can be useful to bring an economy from a depressed state (!). The rough idea being that a relaxation of fiscal policy leads to a higher output and the increase in economic activity leads to a stabilization of public debt/gdp ratio.

This condition is valid as long as (in the authors’ terminology):

(click to enlarge)

The interesting thing about this is that the authors suggest that even if r > g, it is possible for the public debt/gdp to remain sustainable under certain conditions.

I won’t have more to say on this because it uses a standard one-period analysis but the fact that some mainstream authors seem to understand the fiscal policy dynamics better is encouraging.

Of course, this result was known to Post Keynesians – Wynne Godley and Marc Lavoie showed this in this paper Fiscal Policy In A Stock-Flow Consistent (SFC) Model (pay-walled, for the working paper click here).

Arguing that their “… conclusions conflict with those of the “new consensus,” which holds that a correct setting of interest rates is the necessary and sufficient condition for achieving noninflationary growth at full employment, leaving fiscal policy rather in the air.”, they also derive a result for a closed economy:

It is usually asserted that, for the debt dynamics to remain sustainable, the real rate of interest must be lower than the real rate of growth of the economy for a given ratio of primary budget surplus to gdp. If this condition is not fulfilled, the government needs to pursue a discretionary policy that aims to achieve a sufficiently large primary surplus. We can easily demonstrate that there are no such requirements in a fully consistent stock-flow model such as ours.

The G&L style of modeling is extremely useful because it gives great attention to stocks and flows so that no errors creep in. The result is surprising the first time one hears this because it goes against intuition. This can be seen by thinking of the interest payments of the government as income for the domestic private sector!

So no conditions such as r < g!

Open Economy Debt Dynamics

For open economies, G&L are also able to construct select scenarios where a debtor nation can be indebted to the rest of the world without the nation’s debt (which is different from public debt) increasing relative to gdp forever. (Of course by no means proving/implying it for all possible scenarios).

So let us consider the debt dynamics equation (see A Practical Guide To Public Debt Dynamics, Fiscal Sustainability And Cylical Adjustment of Budgetary Aggregates from the IMF, which can be equally applied to the open economy case)

where d is “external debt” and pb is the primary balance of the current account balance. (The expressions are relative to gdp)

 

Suppose the government and the central bank want to restrict external debt to 50% of gdp – with the view that foreigners may consider moving above it as unsustainable. Assume growth is 3.5% and effective interest rate paid on liabilities to foreigners is 1%. Then the tolerable primary deficit of current account balance is 1.25%. This is calculated by setting the left hand side to zero and just plugging the formulas. (See below)

Please note, a higher growth will worsen the external balance so it is not a good argument that growth can lead to a lower debt/gdp ratio.

To summarize, intuitions for a closed economy and the open economy can appear contradictory.

Standard Analysis

I have seen many economists including Post Keynesians (not G&L) take the above equation and interpret it rather differently. So assuming sustainability, a constant primary balance pb implies the debt sustains at

or simply,

A continuous time formulation leads to an equality sign. The above is derived by assuming the debt sustains at d and shuffling the terms in the first equation.

Note: the above is valid only if there a stabilization. Else, in the case where g < r, the above expression gives a negative answer for a negative primary balance – but that is because the derivation assumed sustainability and cannot be used when debt/gdp keeps rising.

This is also written sometimes as

by expanding the denominator of the previous expression using the Taylor Theorem from Mathematics.

This raises a curiosity – how come in the G&L case for the closed economy did the debt sustain even when g > r? That’s because it was a dynamic stock-flow consistent model as opposed to the artificial assumption of a constant deficit used in standard analysis such as the third equation above.

Nonetheless the above analysis shows that for a constant deficit (though artificial), debt sustains as per the equation (assuming growth and the rate of interest paid are constant as well!)

Back to Open Economies

Moving to the open economy case, where debt and deficit denote the external debt and the current account deficit, the above shows that if the primary deficit is restricted somehow to say 5% of gdp and the differential between growth and interest rate is 2%, the external debt sustains at 250% of gdp.

This should be seen as a restriction. Instead some/many Post Keynesians just state it is sustainable. A higher growth rate will increase the deficit (current account) and the debt-dynamics can make the whole process unsustainable. So one needs to model how fiscal policy itself affects the current account deficit rather than keeping it a constant relative to gdp.

This is the reason many nations find themselves troubled by the external sector.

This can be seen for the case of the United States. A huge relaxation of fiscal policy will bring back the current account deficit to 6% of gdp (and rising) and put the world on an unsustainable path. What the United States needs to do is ask its trading partners to expand domestic demand by fiscal expansion and achieve higher growth so that it itself can achieve a higher growth rate due to the extra space created for fiscal policy.

More generally, we need a concerted action!

For a related analysis see Dean Baker’s recent analysis on the trade deficit being America’s fundamental imbalance: The Iron Grip of Accounting Identities

Summary

There is no condition such as if r <g, debt is sustainable. Debt can keep rising relative to gdp simply because deficit keeps rising.

The condition r > g can be useful in studying certain circumstances for analysis.

Kalecki’s Profit Equation

Posted on by
Leave a reply

In my post The Transactions Flow Matrix, I went into how a full transactions flow matrix can be constructed using a simplified national income matrix. Let us reanalyze the latter. The following is the same matrix with some modifications – firms retain earnings and there are interest payments.

FU is the undistributed profits of firms. From the last line we immediately see that

SAVh + FU – If – DEF = 0

or that

FU = If + DEF – SAVh

This is Kalecki’s profit equation which says among other things that firms’ retained earnings is related to the government deficit! The equation appears in pages 82-83 of the following book by Michal Kalecki:

click to view on Google Books

In their book Monetary Economics, Wynne Godley and Marc Lavoie say this in a footnote:

Note that neo-classical economists don’t even get close to this equation, for otherwise, through equation (2.4), they would have been able to rediscover Kalecki’s (1971: 82–3) famous equation which says that profits are the sum of capitalist investment, capitalist consumption expenditures and government deficit, minus workers’ saving. Rewriting equation (2.3), we obtain:

FU = If + DEF − SAVh

which says that the retained earnings of firms are equal to the investment of firms plus the government deficit minus household saving. Thus, in contrast to neo-liberal thinking, the above equation implies that the larger the government deficit, the larger the retained earnings of firms; also the larger the saving of households, the smaller the retained earnings of firms, provided the left-out terms are kept constant. Of course the given equation also features the well-known relationship between investment and profits, whereby actual investment expenditures determine the realized level of retained earnings.

The above can of course also be written as:

I = SAVh + SAVf + SAVg = SAV

if one realized that the retained earning of firms is also their saving:

SAVf = FU

Business accountants know the connection between retained earnings and shareholders’ equity and in our language – which is that of national accountants/2008 SNA – it adds to their net worth just like household saving adds to their net worth.

Assuming away capital gains, we know from many posts that:

Change in Net Worth = Saving

Where do we find the undistributed profits in the Federal Reserve’s Flow of Funds Statistic Z.1?

In Table F.102, there’s an item called “Total Internal Funds”:

(click to expand)

Ya He Averiguado Lo Que Es La Economía

Posted on by
Leave a reply

– es la ciencia de confundir los stocks con los flujos.

Or …

I have found out what economics is; it is the science of confusing stocks with flows.

– A verbal statement by Michal Kalecki, circa 1936, as cited by Joan Robinson, in ‘Shedding darkness’, Cambridge Journal of Economics, 6(3), September 1982, 295–6 and quoted in Monetary Economics written by Wynne Godley and Marc Lavoie. And that’s how Chapter 1 starts and the authors aim to straighten out the messes of the profession and set the confusions straight!

Javier López Bernardo has translated the book in Spanish and here’s the publisher’s website for the book. The publisher’s preview is here.

The author has also implemented the models in Microsoft Excel and you can find them here. I try do that myself but it’s not in such a neat form.